|
|
Definition of the Calendar
The Meyer-Palmen Solilunar Calendar (MPSLC) designates days by means of dates consisting of four numbers:
where the day number ranges from 1 through 31, the month number ranges from 1 through 13, the year number ranges from 1 through 60 and the cycle number is an integer (-2, -1, 0, 1, 2, 3, ...). This calendar measures time in cycles of sixty years: the first year of a cycle is year 1 and the last is year 60. Each year has 12 or 13 months, and each month has 29, 30 or 31 days. A typical date is 89-49-06-09, the 9th day in the 6th month of the 49th year in cycle 89.
Most years have twelve months, but some years have thirteen. A year with thirteen months is known as a "long year". All odd-numbered months have 29 days and all even numbered months have 30 days, except that the 13th month in a long year has either 30 or 31 days.
The months are named after distinguished persons in the history of calendars, mathematics, cosmology and astronomy:
| Month number | Month name | Number of days | Month number | Month name | Number of days | |
| 1 | Aristarchus | 29 | 7 | Galileo | 29 | |
| 2 | Bruno | 30 | 8 | Hypatia | 30 | |
| 3 | Copernicus | 29 | 9 | Ibrahim | 29 | |
| 4 | Dee | 30 | 10 | Julius | 30 | |
| 5 | Eratosthenes | 29 | 11 | Khayyam | 29 | |
| 6 | Flamsteed | 30 | 12 | Lilius | 30 | |
| 13 | Meton | 30 or 31 |
|
(i) Year y in cycle c is a long year if and only if ((60.c + y) . L) mod Y < L
(ii) If year y in cycle c is a long year then the month of Meton has 31 days (rather than 30) if and only if ( [((60.c + y) . L) / Y] . M) mod L < M where [...] means truncation to the next lower integer.
A sequence of 114 consecutive cycles, beginning with a cycle whose number is exactly divisible by 114, is called an "era". Era 0 begins with 000-01-01-01.
The MPSLC is related to the Julian day number system (and thus to the Common Era Calendar) as follows: 000-01-01-01 MP, the first day of the first month of the first year in cycle 000 corresponds to Julian day number 207,227 (-4145-04-08 CE).
This completes the definition of the Meyer-Palmen Solilunar Calendar.
This calendar was invented by Peter Meyer in March 1999 starting from a general form of the above rule for long years etc. devised by Karl Palmen and posted to the CALNDR-L mailing list on 1999-02-24.
Properties of the Calendar
Here is a list of some properties of the MPSLC. Some of these are discussed further in subsequent sections.
- The structure of the MPSLC repeats after 114 cycles (one era). In one era there are exactly 6840 years, of which 2519 are long years. Of these long years, exactly 1328 have a month of Meton with 31 days.
- The number of years in an era, 6840, is divisible by 32 numbers less than 200.
- For the nth long year (where the first long year of cycle 000 is year 3,
beginning on 000-03-01-01 MP) the expression [((60.c + y) . L) / Y]
evaluates to n. Hence the expression in part (ii) of the rule
can be simplified to (n.M) mod L < M, if n is known.
- Approximately 36.83% of years are long years, and approximately 52.72% of long years have a month of Meton with 31 days.
- The date 1795-03-20 CE, at which a vernal equinox and a new (dark) moon occurred together, corresponds to 099-01-01-01 MP, the first day of cycle 099.
- The date 1999-03-17 CE corresponds to 102-25-01-01 MP, and the date 1999-08-11 CE (the date of a total solar eclipse) corresponds to 102-25-06-01 MP.
- The first day of an era is always a Sunday. Era 0 begins at 000-01-01-01, which corresponds to Sunday, -4145-04-08 CE. The next era begins at 114-01-01-01, which corresponds to Sunday, 2695-04-07 CE. The following era begins at 228-01-01-01, which corresponds to Sunday, 9535-04-07 CE.
- In one era there are exactly 2,498,258 days.
- The number of days in an era is a multiple of 7, and in one era there are 356,894 weeks. Since both weeks and eras begin on a Sunday, the 356,894 weeks fit exactly into one era.
- The average length of the MPSLC year is 2,498,258 / 6840 =
365.24239766 MPSLC days, which lies within the expected range during the next 1000 years of 365.24236 to 365.24243 Universal Days for the vernal equinox year.
- In one era there are exactly 84,599 months.
- The average length of the MPSLC month is 2,498,258 / 84,599 = 29.530585468 MPSLC days, which lies within the expected range during the next 1000 years of 29.530583 to 29.530590 Universal Days for the observed (not TAI) synodic month.
- The earliest that a total lunar eclipse during 1600-2500 CE occurs is 4 p.m. (GMT) on a 14th of the MPSLC month, and the latest is 3 a.m. (GMT) on an 18th. Most such eclipses occur on the 15th or the 16th of the month. The average difference from midnight of the 15th/16th is about twelve hours.
- All New Year's Days in the MPSLC occur from 6 March through 7 April of the Common Era Calendar and are evenly distributed over this range of dates. The mean date in this range, 22 March, is close to the usual date of the vernal equinox, 20 or 21 March.
For interesting comments on some mathematical properties of the MPSLC see Karl Palmen's Some Properties of the Meyer-Palmen Solilunar Calendar.
Correlation with the Common Era Calendar
In order to relate the Meyer-Palmen Solilunar Calendar to the Gregorian (or Common Era) Calendar it is sufficient to associate a date in the MPSLC with a Julian day number. This then establishes a one-to-one correspondence between MPSLC dates and Julian day numbers. Since there is already an established one-to-one correspondence between Julian day numbers and dates in the Common Era Calendar, this establishes a correlation between the MPSLC and the CE Calendar.Thus the correlation question may be reduced to: Which Julian day number corresponds to the "first" day in the MPSLC, 000-01-01-01? This number is termed "the MPSLC base Julian day number", or "base number" for short.
It is desirable to use a base number far enough into the past that most dates in the MPSLC for past events of interest will have a cycle number which is positive. For this reason I considered only Julian day numbers in the range 0 through c. 625,000 (-3001-02-02 CE). (For an explanation of negative years see Astronomical Year Numbering.) After an examination of various possibilities I arrived at the base number given above — 207,227 — which means that 000-01-01-01 MP is equivalent to -4145-04-08 CE, or 8 April 4146 B.C.
Using this base number we obtain the following correspondences between dates in the MPSLC and dates in the CE Calendar:
CE Calendar ----- Meyer-Palmen Solilunar Calendar ----- Julian day number -4713-11-24 -10-33-09-21 Monday, Ibrahim 21, -10-33 0 -4713-11-25 -10-33-09-22 Tuesday, Ibrahim 22, -10-33 1 -4713-11-26 -10-33-09-23 Wednesday, Ibrahim 23, -10-33 2 -4713-11-27 -10-33-09-24 Thursday, Ibrahim 24, -10-33 3 -4713-11-28 -10-33-09-25 Friday, Ibrahim 25, -10-33 4 -4713-11-29 -10-33-09-26 Saturday, Ibrahim 26, -10-33 5 -4713-11-30 -10-33-09-27 Sunday, Ibrahim 27, -10-33 6 -4713-12-01 -10-33-09-28 Monday, Ibrahim 28, -10-33 7 -4713-12-02 -10-33-09-29 Tuesday, Ibrahim 29, -10-33 8 -4713-12-03 -10-33-10-01 Wednesday, Julius 1, -10-33 9 -4713-12-04 -10-33-10-02 Thursday, Julius 2, -10-33 10 -4713-12-05 -10-33-10-03 Friday, Julius 3, -10-33 11
CE Calendar ----- Meyer-Palmen Solilunar Calendar ----- Julian day number
1899-12-27 100-45-10-26 Wednesday, Julius 26, 100-45 2,415,016
1899-12-28 100-45-10-27 Thursday, Julius 27, 100-45 2,415,017
1899-12-29 100-45-10-28 Friday, Julius 28, 100-45 2,415,018
1899-12-30 100-45-10-29 Saturday, Julius 29, 100-45 2,415,019
1899-12-31 100-45-10-30 Sunday, Julius 30, 100-45 2,415,020
1900-01-01 100-45-11-01 Monday, Khayyam 1, 100-45 2,415,021
1900-01-02 100-45-11-02 Tuesday, Khayyam 2, 100-45 2,415,022
1900-01-03 100-45-11-03 Wednesday, Khayyam 3, 100-45 2,415,023
1900-01-04 100-45-11-04 Thursday, Khayyam 4, 100-45 2,415,024
1900-01-05 100-45-11-05 Friday, Khayyam 5, 100-45 2,415,025
CE Calendar ----- Meyer-Palmen Solilunar Calendar ----- Julian day number
2100-09-29 104-06-07-26 Wednesday, Galileo 26, 104-06 2,488,341
2100-09-30 104-06-07-27 Thursday, Galileo 27, 104-06 2,488,342
2100-10-01 104-06-07-28 Friday, Galileo 28, 104-06 2,488,343
2100-10-02 104-06-07-29 Saturday, Galileo 29, 104-06 2,488,344
2100-10-03 104-06-08-01 Sunday, Hypatia 1, 104-06 2,488,345
2100-10-04 104-06-08-02 Monday, Hypatia 2, 104-06 2,488,346
2100-10-05 104-06-08-03 Tuesday, Hypatia 3, 104-06 2,488,347
2100-10-06 104-06-08-04 Wednesday, Hypatia 4, 104-06 2,488,348
2100-10-07 104-06-08-05 Thursday, Hypatia 5, 104-06 2,488,349
Variation of New Year's Day
The rules of the MPSLC for long years, etc., were intentionally designed to minimize the extent of the drift of the calendrical New Year's Day (NYD) with respect to the seasons. We find, in fact, that, as stated above, all NYDs in the MPSLC occur from 6 March through 7 April of the Common Era Calendar.The following table shows the frequency with which NYDs fall on days in March and in April. The first column gives the frequencies for all years in the range 1900 through 2100 CE, the second for years 1500 CE through 2500 CE, and the third for years 0 CE through 4000 CE.
2000CE+/-100 |
2000CE+/-500 |
2000CE+/-2000 |
|
201 NYDs |
1001 NYDs |
4001 NYDs |
|
March 6 |
1, 0.5% |
3, 0.30% |
7, 0.17% |
March 7 |
5, 2.5% |
22, 2.20% |
74, 1.85% |
March 8 |
5, 2.5% |
28, 2.80% |
132, 3.30% |
March 9 |
6, 3.0% |
31, 3.10% |
128, 3.20% |
March 10 |
8, 4.0% |
37, 3.70% |
132, 3.30% |
March 11 |
5, 2.5% |
29, 2.90% |
129, 3.22% |
March 12 |
8, 4.0% |
38, 3.80% |
136, 3.40% |
March 13 |
6, 3.0% |
31, 3.10% |
128, 3.20% |
March 14 |
5, 2.5% |
28, 2.80% |
131, 3.27% |
March 15 |
9, 4.5% |
39, 3.90% |
133, 3.32% |
March 16 |
5, 2.5% |
30, 3.00% |
127, 3.17% |
March 17 |
7, 3.5% |
33, 3.30% |
133, 3.32% |
March 18 |
7, 3.5% |
33, 3.30% |
130, 3.25% |
March 19 |
5, 2.5% |
30, 3.00% |
134, 3.35% |
March 20 |
9, 4.5% |
36, 3.60% |
132, 3.30% |
March 21 |
6, 3.0% |
32, 3.20% |
127, 3.17% |
March 22 |
6, 3.0% |
31, 3.10% |
131, 3.27% |
March 23 |
8, 4.0% |
37, 3.70% |
136, 3.40% |
March 24 |
5, 2.5% |
27, 2.70% |
129, 3.22% |
March 25 |
7, 3.5% |
34, 3.40% |
132, 3.30% |
March 26 |
7, 3.5% |
37, 3.70% |
128, 3.20% |
March 27 |
5, 2.5% |
30, 3.00% |
131, 3.27% |
March 28 |
8, 4.0% |
34, 3.40% |
131, 3.27% |
March 29 |
5, 2.5% |
30, 3.00% |
134, 3.35% |
March 30 |
6, 3.0% |
32, 3.20% |
128, 3.20% |
March 31 |
10, 5.0% |
40, 4.00% |
137, 3.42% |
April 1 |
4, 2.0% |
27, 2.70% |
125, 3.12% |
April 2 |
8, 4.0% |
35, 3.50% |
132, 3.30% |
April 3 |
7, 3.5% |
34, 3.40% |
134, 3.35% |
April 4 |
4, 2.0% |
27, 2.70% |
128, 3.20% |
April 5 |
10, 5.0% |
39, 3.90% |
134, 3.35% |
April 6 |
1, 0.5% |
17, 1.70% |
95, 2.37% |
April 7 |
3, 1.5% |
10, 1.00% |
23, 0.57% |
From this we see that all dates in the range 6 March through 7 April, except for the first two dates and the last two, occur with a frequency of between 3.12% and 3.42% (during the period 0 through 4000 CE), so clearly the NYDs are distributed fairly evenly throughout the range of dates 8 March to 5 April, with lesser frequencies for the preceding and succeeding two dates.
This table was produced by means of the program NYD.C.
We can get a better view of this data by graphing it, as follows:
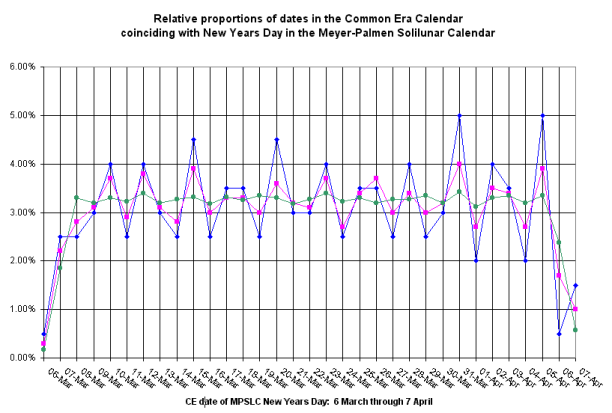
The blue line joins the relative proportions of New Years Day dates for all years in the range 1900 CE through 2100 CE (201 cases), the mauve line for years 1500 CE through 2500 CE (1001 cases), and the green line for years 0 CE through 4000 CE (4001 cases). It can be seen that the variation in the data decreases as the number of cases increases.
Date Conversion Software
There is Windows software for converting between dates in the Common Era Calendar and dates in the Meyer-Palmen Solilunar Calendar (and a variety of other dates). For further details see Lunar Calendars and Eclipse Finder.
| MPSLC Date Conversion Software | |
| Some Properties of the Meyer-Palmen Solilunar Calendar | |
| Calendar Studies | Home Page |